Population Balance Modelling
Population Balances are a widely used tool in engineering, with applications including crystallisation, pharmaceutical manufacture, pollutant formation in flames and growth of microbial and cell populations [1]. Wherever the interaction of a large number of particles (be they gas-phase nano-particles, liquid droplets in liquid-liquid dispersions or solid powder agglomerates) is studied, solution of the population balance equation is necessary to determine the properties of the resulting product and its dependence on processes such as coalescence, breakage and surface growth.
The form of the population balance equation (PBE) is as follows:
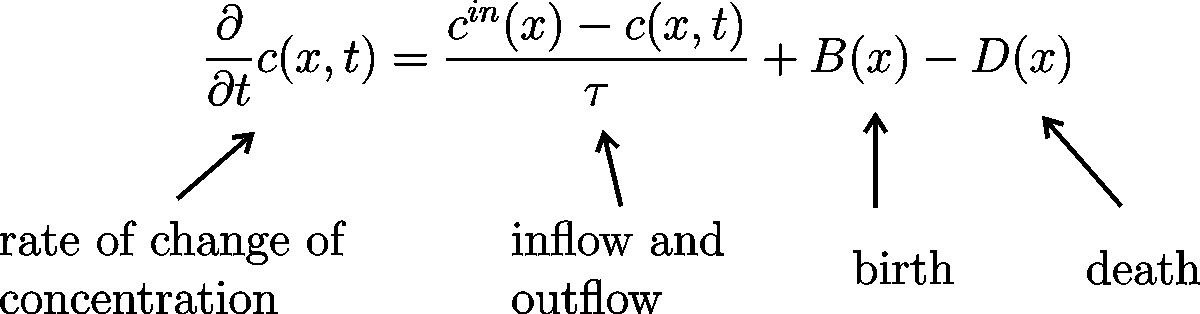 |
| The Population Balance Equation |
The birth and death terms are frequently integral functions of the whole population, so a complicated integro-differential equation must be solved numerically to resolve the properties of the whole population. There exist well-established deterministic methods that can be used when there is only one particle property (x) of interest. In the multi-variate case however, when two or more properties (such as size, shape, composition etc.) are introduced, the efficiency of deterministic methods suffers and stochastic methods are an attractive alternative.
In this project we develop new numerical methods for the solution of multidimensional population balances with and without spatial dependence. These methods are based on stochastic particle methods which have been recently developed by Eibeck and Wagner [2,3]. Currently this work is carried out in cooperation with Dr Wolfgang Wagner from WIAS, Berlin and Dr James Norris from DPMMS in Cambridge.
References
[1] Ramkrishna D. (2000) Population Balances. Theory and Applications to Particulate Systems in Engineering, Academic Press, San Diego.
[2] Eibeck A. and Wagner W. (2000) SIAM J. Sci. Comput., 22(3), 802-821
[3] Eibeck A. and Wagner W. (2001) Ann. Appl. Probab., 11(4), 1137-1165