Extension of moment projection method to the fragmentation process
- The moment projection method (MPM) is extended to include the fragmentation process
- MPM is tested for symmetric fragmentation and erosion fragment distribution functions and shows high accuracy
- MPM is also able to accurately simulate the combined processes of inception, growth, shrinkage, coagulation and fragmentation
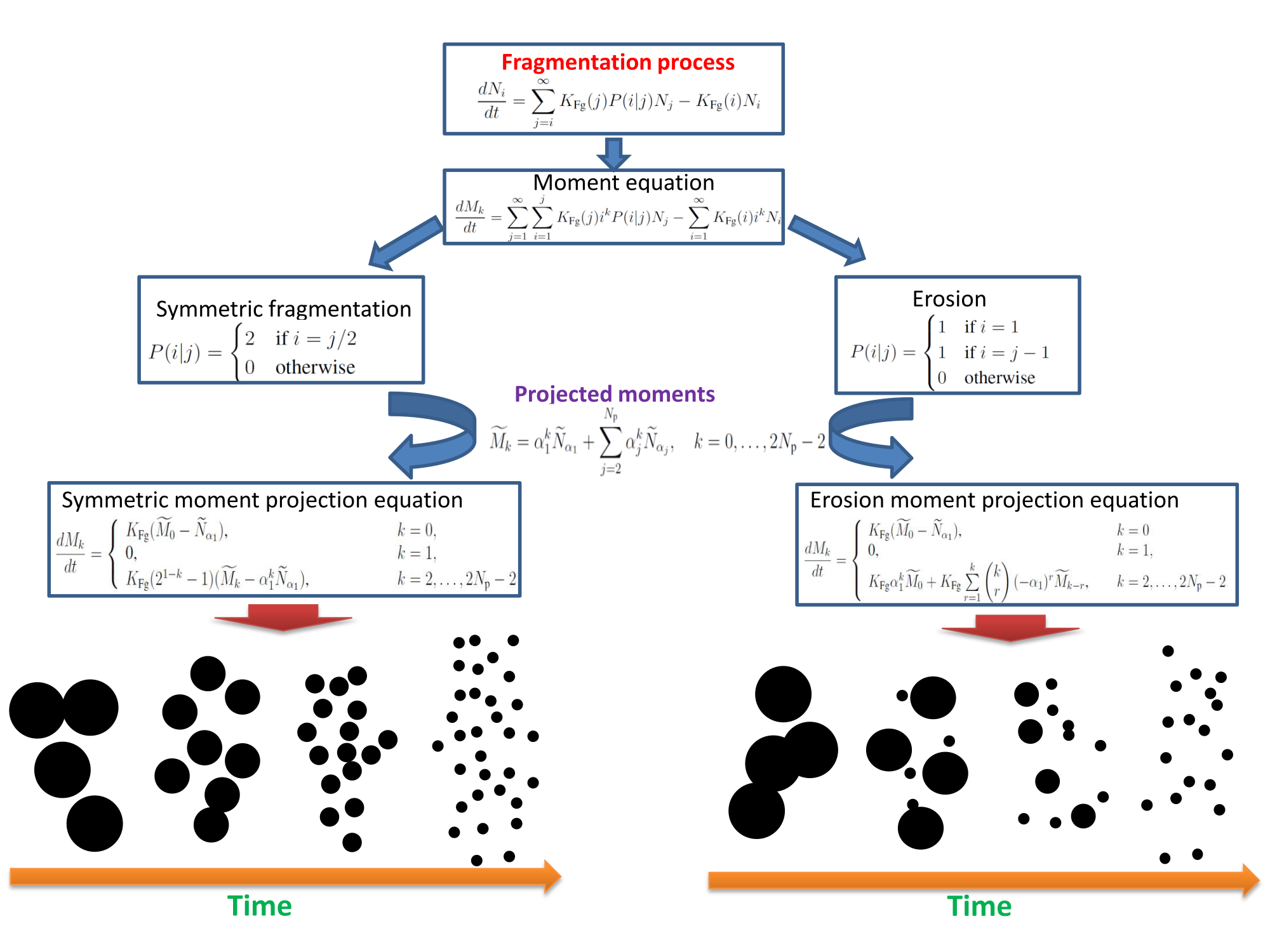The method of moments is a simple but efficient method of solving the population balance equation which describes particle dynamics. Recently, the moment projection method (MPM) was proposed and validated for particle inception, coagulation, growth and, more importantly, shrinkage; here the method is extended to include the fragmentation process. The performance of MPM is tested for 13 different test cases for different fragmentation kernels, fragment distribution functions and initial conditions. Comparisons are made with the quadrature method of moments, hybrid method of moments and a high-precision stochastic solution calculated using the established direct simulation algorithm and advantages of MPM are drawn.
- This paper draws from preprint 172: Extension of moment projection method to the fragmentation process
- Access the article at the publisher: DOI: 10.1016/j.jcp.2017.01.045
Associated Themes:
