Technical Report 186, c4e-Preprint Series, Cambridge
The Mathematical Foundations of An Efficient Stochastic Algorithm For Solving Population Balance Equations
Reference: Technical Report 186, c4e-Preprint Series, Cambridge, 2017
- The mathematical foundations of the single particle method are developed.<
- The convergence and numerical properties of the method are investigated in detail.<
- Additional jump processes are introduced to improve the convergence character.<
- The method is extended to spatially dependent problems with non-uniform velocities.<
- Parameter studies are performed in order to justify the use of the method in CFD applications.
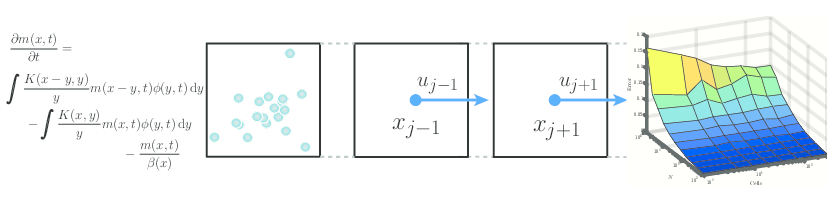 This paper develops for the first time the mathematical foundations of an efficient stochastic algorithm for solving steady-state population balance equations, which is suitable for coupling to computational fluid dynamics codes. The properties and performance of the algorithm are charted using a systematic comparison with an existing stochastic algorithm. The algorithm is extended to 1D geometries with non-uniform flows. The method is enhanced by the inclusion of additional stochastic jump processes to improve its convergence characteristics and studies are performed in order to establish values of key parameters rendering the method suitable for CFD applications.
This paper develops for the first time the mathematical foundations of an efficient stochastic algorithm for solving steady-state population balance equations, which is suitable for coupling to computational fluid dynamics codes. The properties and performance of the algorithm are charted using a systematic comparison with an existing stochastic algorithm. The algorithm is extended to 1D geometries with non-uniform flows. The method is enhanced by the inclusion of additional stochastic jump processes to improve its convergence characteristics and studies are performed in order to establish values of key parameters rendering the method suitable for CFD applications.
PDF (1.5 MB)